area of a sector?" width="1057" height="599" />Here we will learn how to calculate the area of a sector including how to identify a sector of a circle, form and use the formula for the area of a sector of a circle and calculate the area of a sector in various scenarios.
There are also area of a sector circle worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
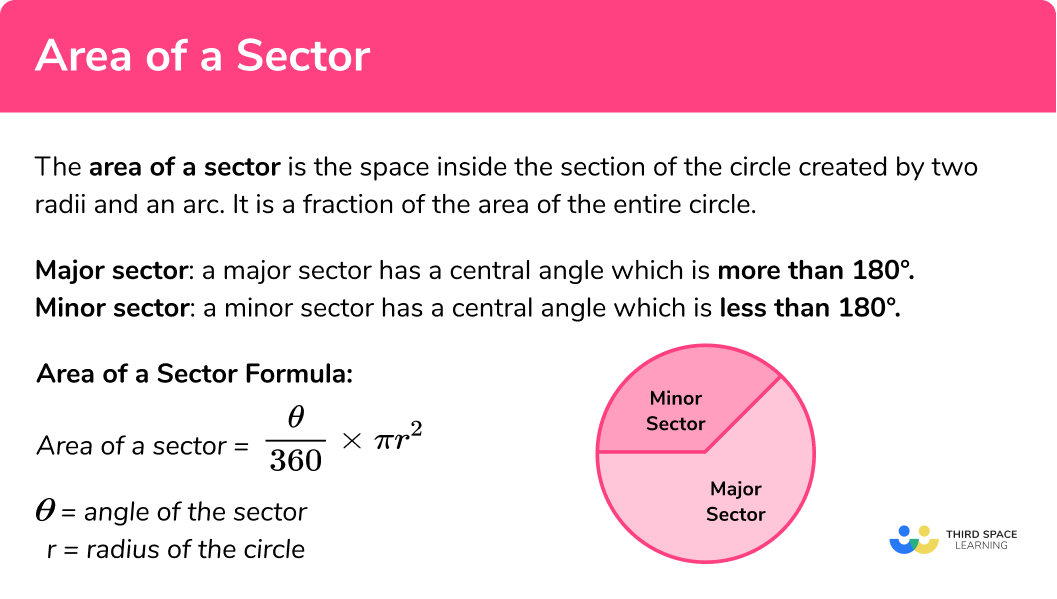
The area of a sector is the space inside the section of the circle created by two radii and an arc. It is a fraction of the area of the entire circle.
Major sector: a major sector has a central angle which is more than 180° .
Minor sector: a minor sector has a central angle which is less than 180° .
Area of a sector =\frac \times \pi r^
θ = angle of the sector
r = radius of the circle
area of a sector?" width="1057" height="599" />
In order to find the area of a sector you need to be able to find the area of a circle. This is because the area of a sector is part of the total area of the circle.
‘How much’ of the circle is decided by the angle created by the two radii.
The sum of the angle around a point is equal to 360° .
Therefore, the area of a sector is the fraction of the full circle’s area.
The angle is out of 360 or \frac , where θ represents the angle, so we can multiply this by the area of the circle to calculate the area of a sector.
NOTE: At GCSE, all angles are measured in degrees. Make sure that your calculator has a small ‘d’ for degrees at the top of the screen rather than an ‘r’ for radians – these are not used until A Level.
Area of a sector formula:
Area of a sector = \frac \times \pi r^
θ = angle of the sector
r = radius of the circle
In order to solve problems involving the area of a sector you should follow the below steps:
